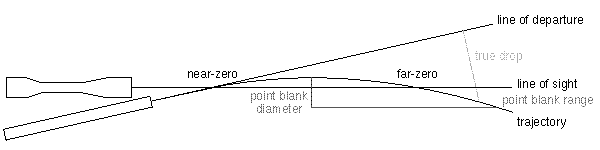
A common misconception is that a bullet will rise for a while after firing from a horizontal barrel. In fact, a bullet fired from a horizontal barrel will fall towards the earth at the same rate as a bullet dropped from the hand at the instant of firing. The origin of the misconception is that in order to have the bullet cross the line of sight downrange, the barrel and sights must be misaligned such that the barrel is angled upwards relative to the sights. As a result, the bullet is "lobbed" towards the target and crosses the line of sight at two points, the near-zero and the far-zero. This is illustrated by the following figure:

The angles in the figure have been grossly exaggerated for clarity. It can be seen that the bullet is rising as it crosses the near-zero and falling as it crosses the far-zero. Unless you sight your rifle in at very short range, it is likely that the x-ring of your target is at the far-zero of the trajectory. Also illustrated are the differences between the line of departure (which extends from the barrel), the line of sight (which extends from the scope or other sighting apparatus), and the trajectory.
The WBC calculates the drop from line of sight based on a far zero that you set equal to the distance at which you sight in the rifle. The WBC will also tell you the corresponding near-zero.
The Web Ballistics Computer allows you to specify a sight height and adjusts the trajectory accordingly. In the case of optical sights, the sight height is measured from the center of the bore to the center of the objective (front) lens of the sight. Iron sights are measured from the center of the bore to the top of the front sight.
For instance, say you're hunting a deer and you know that its vital area is about 5" across. You look up the ballistics for your factory load and find that the bullet is 1.5" high at 100 yards and is 3.5" inches low at 250 yards. Since you know that the bullet will fall within 5 inches of point of aim at any distance out to 250 yards, this is defined to be the point blank range. Note that the point blank range is not the distance at which the drop is 5 inches because the bullet is above the line of sight earlier in flight. Mine is a conservative definition, allowing you to grossly misjudge the range to the target and still put the bullet within the point blank diameter, regardless of whether you shoot a bullet with a curved or flat trajectory. For extremely curved trajectories, the bullet may exceed the point blank diameter before it reaches the high point of the trajectory, in which case the point blank range will be shorter than the far zero. The Web Ballistics Computer can find the point blank range regardless of the curvature of the trajectory. It doesn't assume that you're aiming at the center of a "circle of uncertainty", just that the bullet will strike within a given distance from the point of aim. Beyond point blank range, you'll have to take into account the trajectory of the bullet in order to hit the zone you've specified by "holding over" the required amount.
The Web Ballistics Computer can account for the effects of altitude and temperature on the flight of the bullet but it cannot account for the fact that powder burns more slowly on cold days or that your barrel is dirty. For this reason, it bears repeating that you should test fire your rifle at various distances to determine its performance (and yours) before a hunt.
If you don't know the ballistic coefficient of your bullet, the Web Ballistic Computer will allow you to choose from one of four shape classes. It will then calculate an approximate ballistic coefficient based on the bullet shape, diameter, and weight.